


Lunar Eclipse
by Peter Alway
| A lunar eclipse happens when the Moon passes into the Earth's Shadow. Lunar eclipses are common. On average they happen twice a year. But only half the world gets to view any Lunar eclipse, and some eclipses--penumbral eclipses--are hardly noticeable. So it was a special opportunity to see a lunar eclipse during a semester I'm teaching Introductory Astronomy at Schoolcraft College. I was pleased to see that most had produced respectable sketches showing the curve of the Earth's shadow. This meant we had the data to measure the relative sizes of the Earth and Moon, just as the ancient Greek astronomer Aristarchus had done 2300 years ago. | |
| Lowbrow Home Page | |
| Club Information | |
| Lowbrow Astro Pictures | |
| Openhouses | |
| McMath 24" Telescope | |
| Astronomy Links | |
| Search | |
| Observer's Guide |
My Astronomy Pages:
 |  |  |  |  |
The Path of the Moon
The Moon orbits the earth roughly every 30 days or so. the diagram below shows the path, and the illumination of the Moon that causes its phases.

From this drawing, you would think that the Moon would end up in the Earth's shadow once a month. But the Moon's orbit is not exactly in the plane of the Earth's orbit around the Sun. As a result, the Moon usually passes above or below the Earth's Shadow. But twice a year, the plane of the Earth's orbit and the plane of the Moon's orbit line up so that a full Moon can pass through the Earth's shadow. Depending on when the full Moon occurs near those alignments, there may be a total lunar eclipse, in which the Moon is completely immersed in darkness, a partial lunar eclipse, when part of the Moon is in total darkness, or a penumbral eclipse, when the Moon just skims the fuzzy outer part of the Earth's shadow. The time of full Moon also determines where in the world it the eclipse will be seen.
The US Naval Observatory is the authoritative source for eclipse predictions. Another site a NASA's Goddard Spaceflight Center has some nice graphical stuff on coming eclipses.
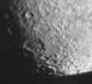
A total lunar eclipse is a neat sight. The moon turns dark, with a warm tinge of brownish grey, orange, or blood red. This is caused by sunlight sneaking around the Earth via refraction in the Earth's atmosphere. Just as the setting sun is red or orange, the light reaching the moon takes on a reddish tint.
Here is a picture of January's total eclipse by Trygve Lode He just pointed his digital camera up--no telescope involved:

But a partial lunar eclipse, or the partial phase of a total lunar eclipse, presents us with a unique opportunity. We can see ourselves in silhouette. The ancient Greek astronomer Aristarchus noticed that the edge of our shadow was always the same gently curved arc. If the Earth were flat, the shadow would have at least a flat side whe an eclipse occured at sunrise or sunset. If the Earth were anything but a round ball, our shadow would look different from eclipse to eclipse. Aristarchus proved the earth was round 1800 years before Columbus.

Finding the relative sizes of the Earth and Moon
If you could just measure the curve of that silhouette, you could see how big the Earth is compared to the Moon. To do so, you need a picture of the Moon during a partial lunar eclipse, or during the partial phase of a total lunar eclipse. On January 20, 2000, the Moon rode its orbit into the Earth's shadow. I wanted the eclipse tobe a quality astronomica experience for my students, but it the eclipse was not a class night, and with clouds and snow looming, I didn't want to drag my students (or myself) to school for a session running from 9:00 PM to midnight or later. So I issued the students an obserivng form with 1.5" circles to fill with drawings of the eclipse. I instructed them to take repeated observations at regular intervals, especially during the partial phases.
The weather was indeed nasty. Not only did couds move in to southeastern Michigan, but so did snow. Remarkable, much of the eclipse was visible through falling snow. I was able to make eight obserations through holes in the clouds, with snow falling on my binoculars and my observing form.
After midnight, the sky began to clear. I was able to make five more sketches before the thought of getting up at 5:30 for work sent me to bed.
(Click the thumbnails for my sketches scanned in at 50 pixels per inch)
When class met again the following Monday, almost all of the sudents reported observing the eclipse. Most had managed to sketch the eclipse on their forms, using either naked eye or binocular observations. Most of the students sucsessfully sketched the partial phase of the eclipse, including the curve of the shadow. The sutedents' forms look very much like my own. Collectively, we would be able to find the size of the Earth compared to the Moon.
There are a good number of ways of measuring a circular arc: compass-and-straightedge construction, measurement of sagitta, and curve fitting come to mind. But I settled on a more direct method--comparison with a series of circles printed on a transparency. I drew up a series of concentric circles marked in units of 1.5", and had a dozen copies made on transparency material.

(click the tumbnail for a copy scanned in a 50 pixes per inch to match the sketches)
At the next session, each student laid the transparency over their drawings, and read off the diameter of the Earth's inner shadow (umbra) from the circle that most closely matched the curve of the Earth's shadow.
Here is one of my inidvidual sketches:

Here's the sketch with the overlay with my best match of the shadow and a circle:
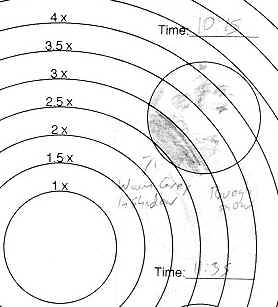
Note that my best match was with the 3x circle--showing the Earth's inner shadow was about three times the size of the Moon. Students' measurments varied from 1.5 x to 7x.

Each student averaged his or her shadow diameters and reported them to me. I also included my average. We had about 20 values, and they averaged out to about 2.8. This means that the sharp inner shadow of the Earth is about 2.8 times the diameter of the Moon.
But the inner shadow of the Earth is smaller than the Earth itself. The actual size of the Earth is midway between the size of the inner shadow (Umbra) and the outer, fuzzy shadow (penumbra)
How wide is the penumbra? It's hard to see the width on the Moon, becuse the outer edge is imperceptible. But it turns out that the angular size of the penumbra is the angular size of the Sun. And the angular size of the Sun (1/2 degree) is almost exactly the angular size of the Moon. So the Penumbra of the Earth's shadow spreads out one Moon diameter on either side of the Umbra.
So the total diameter of the Earth's shadow is the size of the umbra + two Moon diameters, or 2.8 + 2 = 4.8 Moon diameters.
The Earth's diameter is midway between the diameter of the umbra (2.8 Moon diameters) and the diameter of the penumbra (4.8 moon diameters). So we have measured that the Earth is 3.8 times the size of the Moon. The "textbook" number is 3.7.
You could try this measurement with eclipse photos arond the net--but you'll have to scale the overlay to the photos, or scale the photos to your overlay. Here's another of Trygve Lode's photos of the January eclipse, showing the curved shadow of the Earth as the Moon emerged into the light.
Lowbrows Club-Info Lowbrow-Pictures Openhouses Telescope Links Search Observer's-Guide
send e-mail to the author of this page, Peter Alway <petealway@aol.com>