
Gravity, Part 3: Space Travel.
by Dave Snyder
Printed in Reflections: July, 2006.
In part two of this article
(“Gravity, Part 2: Newton, Hooke, Halley and the Three Body Problem,” by Dave Snyder, April, 2006),
I started a discussion of Newton’s Theory of Gravity. I explained how, over the years, astronomers and mathematicians developed methods for predicting motions of the planets, comets and other celestial bodies.
But that isn’t the whole story.
Over the past 50 years we’ve sent numerous spacecraft to the moon and planets. If we want spacecraft to reach their destination, careful planning is needed. The techniques described in part two are helpful when planning space missions, but not sufficient.
Going from A to B
Generally space missions go from point A to point B using a limited amount of fuel. In the past most space missions have used Kepler orbits and/or escape trajectories as much as possible. Kepler orbits were described in part two, they are ellipses—staying in such an orbit requires no fuel. An escape trajectory is a parabola or hyperbola that also requires no fuel. In other words we “coast” as much as possible.
But we cannot coast the entire mission; one or more transfers will be needed, and transfers require fuel.
A common transfer is from a Kepler orbit to a larger/smaller Kepler orbit. There are three ways this is done.
1. Hohmann Transfer Orbits.
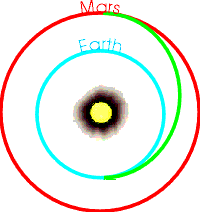 Suppose we are in the orbit “Earth” and we want to be in the orbit “Mars.” We can transfer using what is called a Hohmann orbit (this is a Kepler orbit tangent to the two other orbits—it need not be Mars, it works for most other planets as well). In many cases this is the least expensive way to transfer. However, it has some disadvantages: it is slow and can only be used during specific launch windows, for an Earth-Mars transfer, these windows occur once every two years. For an Earth-Jupiter transfer, the windows occur every 13 months.
Suppose we are in the orbit “Earth” and we want to be in the orbit “Mars.” We can transfer using what is called a Hohmann orbit (this is a Kepler orbit tangent to the two other orbits—it need not be Mars, it works for most other planets as well). In many cases this is the least expensive way to transfer. However, it has some disadvantages: it is slow and can only be used during specific launch windows, for an Earth-Mars transfer, these windows occur once every two years. For an Earth-Jupiter transfer, the windows occur every 13 months.
2. Modified Hohmann Orbits.
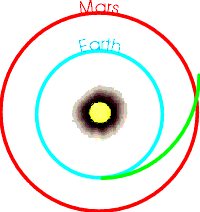 Alternatively we can replace the Hohmann transfer orbit with an orbit that intersects the destination orbit. This is called a modified Hohmann orbit. It is faster than a Hohmann orbit, but requires more fuel.
Alternatively we can replace the Hohmann transfer orbit with an orbit that intersects the destination orbit. This is called a modified Hohmann orbit. It is faster than a Hohmann orbit, but requires more fuel.
3. Gravitational Assist.
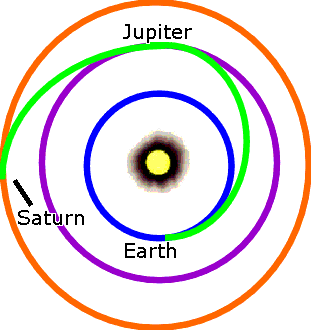 If the spacecraft flies by a planet, the planet will push the spacecraft into a different trajectory. This might either speed the spacecraft up or slow it down (depending the precise circumstances). This is known as gravitational assist (the diagram shows a possible flight from Earth to Saturn using a gravitational assist from Jupiter). This can be faster and/or may use less fuel than is possible with other techniques.
Gravitational assist was first used by Mariner 10 (gravitational assists from Venus and Mercury). It has been used several times since including Voyager (gravitational assists from Jupiter, Saturn, Uranus and Neptune), Cassini (gravitational assists from Venus, Earth and Jupiter) and Messenger (six gravitational assists from Earth, Venus and Mercury).
If the spacecraft flies by a planet, the planet will push the spacecraft into a different trajectory. This might either speed the spacecraft up or slow it down (depending the precise circumstances). This is known as gravitational assist (the diagram shows a possible flight from Earth to Saturn using a gravitational assist from Jupiter). This can be faster and/or may use less fuel than is possible with other techniques.
Gravitational assist was first used by Mariner 10 (gravitational assists from Venus and Mercury). It has been used several times since including Voyager (gravitational assists from Jupiter, Saturn, Uranus and Neptune), Cassini (gravitational assists from Venus, Earth and Jupiter) and Messenger (six gravitational assists from Earth, Venus and Mercury).
If you wish to send the spacecraft out of the solar system, an escape trajectory is needed (as mentioned above, a parabola or hyperbola). Transferring to an escape trajectory takes a lot of fuel (though fuel consumption can be reduced by careful use of gravitational assists).
Using the techniques described so far allow us to flyby a planet. However it is often useful to enter orbit (for observation of the planet and/or to place a lander on the surface). This is often done in two steps.
- An orbital insertion burn can be used to go into orbit, however this typically results in an eccentric orbit around the planet.
- The eccentric orbit can be “circularized” with a rocket burn or, if the planet has an atmosphere, with aero-braking (requires less fuel, but is usually slower than a rocket burn).
Other orbital manipulations are sometimes used, such as changing the inclination (but I wont go into more detail).
Staying Put
So far I’ve talked about going from A to B. Sometimes once we get to a destination, we want to keep stationary. Assuming we can’t just land on a surface, keeping stationary without using large amounts of fuel is tricky, but there is a way to do it involving Lagrangian points. These are five configurations called L1, L2, L3, L4 and L5.
I will be talking about three objects: the Sun, the Earth and a spacecraft (however it doesn’t have to be the Sun and the Earth). You will recall the orbit of the Earth around the Sun is very close to a perfect circle. Now suppose we place a spacecraft so it lies along a line between the Earth and the Sun. If the distances are just right, you get Lagrangian points L1, L2 and L3 (see the diagrams).
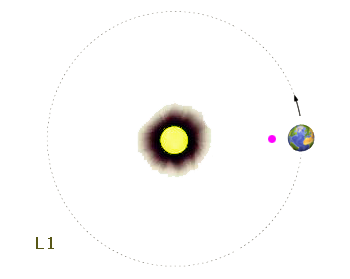

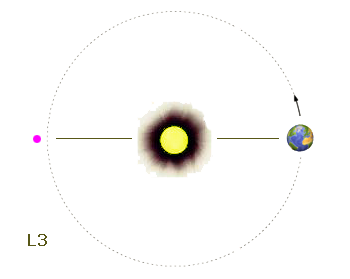
Or you can place the satellite in the same orbit but exactly 60 degrees ahead of the Earth (see diagram L4). Or 60 degrees behind the Earth (diagram L5).
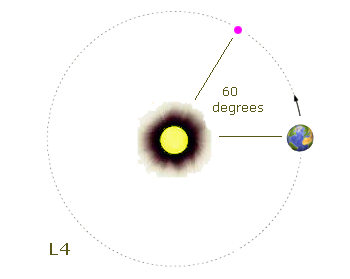
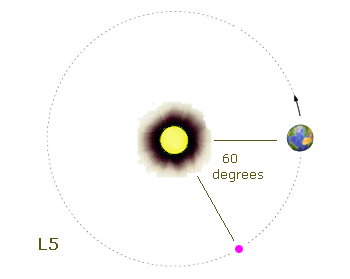
L4 and L5 are stable, once a satellite is at or close to L4, it will tend to stay at or close to L4. The same is true of L5. Thus it possible to park a satellite at either L4 and L5 and it will stay there without expenditure of fuel.
L1, L2 and L3 are metastable. Once a satellite is at or close to L1, it will slowly drift away from L1; the same is true of L2 and L3. Even so this can be a reasonable method for parking a satellite, while the satellite will tend to drift away, this can be controlled with reasonable bursts of fuel.
SOHO (a satellite that observes the sun) uses L1. WMAP (another satellite) uses L2. Other uses of L1 and/or L2 are likely. Since L3 is hidden behind the Sun, it is currently unused and is likely to remain unused. There have been discussions involving space stations at either L4 or L5, however this has not happen yet.
The third object must be much smaller than the other two, but it doesn’t have to be an artificial satellite. It can be an asteroid. If you look at the L4 and L5 points of the Sun and Jupiter, you will find a group of asteroids known as the Apollos. At some point in the past asteroids wandered into these regions, once there they never left. There are also examples involving the moons of Saturn.
The Interplanetary Superhighway
It gets more interesting. If a spacecraft starts near a L1, L2 or L3 point, it is possible to transfer to the “interplanetary superhighway.” This is a set of “tubes” or pathways that allow travel within the solar system with very little fuel.
This technique was first used in 1991. That’s when the first Japanese mission to Moon ran into trouble. Because of a malfunction, soon after launch the spacecraft lost most of the fuel needed to get to the Moon. Using this new approach it was possible to use the existing fuel supply to get to the Moon (however it took three months to do so—compared to a few days using traditional techniques).
I do not have the space to explain the superhighway in any detail, but I will summarize a few main points.
- Trajectories using the superhighway generally require very little fuel, however they take longer than traditional trajectories.
- They are harder to calculate than traditional trajectories. They are constantly changing and require a mathematical technique known as “chaos.” However software has been developed that can calculate these trajectories. You might think chaos is something to be avoided, however we’ve learned that appropriate use of chaos can help in a variety of engineering tasks including controlling spacecraft.
This can be more than just saving a little fuel, it might allow a single mission to visit a variety of destinations in a way that would be impractical with traditional techniques. This was discussed for JIMO (which was a planned tour of Jupiter’s Moons that was cancelled earlier this year).
To make use of the superhighway easier, we could place a permanent space station at one of the metastable points (probably L1). Going between L1 and various other destinations (including points on the Moon or L2) is easier than doing so directly from the Earth.
Given the current budget restrictions that NASA must work with, it is not clear when we will make regular use of the superhighway. Even when we do, it is likely that its primary use will be for unmanned missions. However this is could be another useful technique in planning space missions.
References
------. “Basics of Spaceflight.” http://www2.jpl.nasa.gov/basics/
------. “Cassini-Huygens: Operations-Gravity Assists.”
http://saturn.jpl.nasa.gov/mission/gravity-assists.cfm
------. “MESSENGER—Unlocking the Secrets of Mercury.”
http://www.nasa.gov/mission_pages/messenger/main/index.html
------. “SP-423 Atlas of Mercury—MARINER 10 MISSION AND SPACECRAFT.”
http://history.nasa.gov/SP-423/mariner.htm
------. “Voyager—Science—Planetary Voyage.”
http://voyager.jpl.nasa.gov/science/planetary.html
------. “The Lagrange Points.” http://map.gsfc.nasa.gov/m_mm/ob_techorbit1.html [a discussion of Lagrangian points and a few satellites that have used or might use L1 or L2].
Baez, John. December 19, 2005. “Lagrange Points.” http://math.ucr.edu/home/baez/lagrange.html [contains a good discussion of Lagrangian points, the Trojans and the superhighway].
Karttunen, H. et al. 1996. Fundamental Astronomy. Berlin: Springer, pp. 201-202 [contains a brief discussion of Lagrangian points and the Trojans].
Kaufmann, William J. and Freedman, Roger A. 1999. Universe, Fifth edition. New York: W. H. Freeman and Company, p. 394 [contains a brief discussion of Lagrangian points and the Trojans].
Klarreich, Erica. April 16, 2005. “Navigating Celestial Currents.” Science News. (Volume 167, Number 16, pp. 250-252) [a non-technical discussion of the Interplanetary Superhighway].
Szebehely, Victor G. and Mark, Hans. 1998. Adventures in Celestial Mechanics, 2nd Edition. New York: John Wiley and Sons.
Links
Copyright Info
Copyright © 2015, the University Lowbrow Astronomers. (The University Lowbrow Astronomers are an amateur astronomy club based in Ann Arbor, Michigan).
This page originally appeared in Reflections of the University Lowbrow Astronomers (the club newsletter).
This page revised Tuesday, April 10, 2018 7:08 PM.
This web server is provided by the University of Michigan;
the University of Michigan does not permit profit making activity
on this web server.
Do you have comments about this page or want more information about the club? Contact Us.
 Suppose we are in the orbit “Earth” and we want to be in the orbit “Mars.” We can transfer using what is called a Hohmann orbit (this is a Kepler orbit tangent to the two other orbits—it need not be Mars, it works for most other planets as well). In many cases this is the least expensive way to transfer. However, it has some disadvantages: it is slow and can only be used during specific launch windows, for an Earth-Mars transfer, these windows occur once every two years. For an Earth-Jupiter transfer, the windows occur every 13 months.
Suppose we are in the orbit “Earth” and we want to be in the orbit “Mars.” We can transfer using what is called a Hohmann orbit (this is a Kepler orbit tangent to the two other orbits—it need not be Mars, it works for most other planets as well). In many cases this is the least expensive way to transfer. However, it has some disadvantages: it is slow and can only be used during specific launch windows, for an Earth-Mars transfer, these windows occur once every two years. For an Earth-Jupiter transfer, the windows occur every 13 months.

 Alternatively we can replace the Hohmann transfer orbit with an orbit that intersects the destination orbit. This is called a modified Hohmann orbit. It is faster than a Hohmann orbit, but requires more fuel.
Alternatively we can replace the Hohmann transfer orbit with an orbit that intersects the destination orbit. This is called a modified Hohmann orbit. It is faster than a Hohmann orbit, but requires more fuel.
 If the spacecraft flies by a planet, the planet will push the spacecraft into a different trajectory. This might either speed the spacecraft up or slow it down (depending the precise circumstances). This is known as gravitational assist (the diagram shows a possible flight from Earth to Saturn using a gravitational assist from Jupiter). This can be faster and/or may use less fuel than is possible with other techniques.
Gravitational assist was first used by Mariner 10 (gravitational assists from Venus and Mercury). It has been used several times since including Voyager (gravitational assists from Jupiter, Saturn, Uranus and Neptune), Cassini (gravitational assists from Venus, Earth and Jupiter) and Messenger (six gravitational assists from Earth, Venus and Mercury).
If the spacecraft flies by a planet, the planet will push the spacecraft into a different trajectory. This might either speed the spacecraft up or slow it down (depending the precise circumstances). This is known as gravitational assist (the diagram shows a possible flight from Earth to Saturn using a gravitational assist from Jupiter). This can be faster and/or may use less fuel than is possible with other techniques.
Gravitational assist was first used by Mariner 10 (gravitational assists from Venus and Mercury). It has been used several times since including Voyager (gravitational assists from Jupiter, Saturn, Uranus and Neptune), Cassini (gravitational assists from Venus, Earth and Jupiter) and Messenger (six gravitational assists from Earth, Venus and Mercury).




