


It’s always interesting to compare things. That’s how I got through twelfth grade English class (“Compare and contrast the use of similes in” ... etc., etc.), and comparing galaxies was how I found out that Doug Nell’s eyes are just better than mine, darn it. That’s the thing about comparisons; sometimes they don’t work out the way you’d like. I’m sure that telescope manufacturers feel this way, too. They should, because some products stand up better than others.


It so happened that I needed to get some idea of the point spread function of an 8” Celestron Schmidt Cassegrain. One way to do that is to measure the wavefront error and extrapolate, so I put the telescope into a Fizeau interferometer with a 6” diameter beam, and took a picture of the fringes. I couldn’t get the whole aperture in the beam, so I offset it, edge to edge, and assumed its errors were symmetric (which might or might not be true, depending on the manufacturer, but let’s give them the benefit of the doubt). Then I thought, why not compare the 8” Schmidt-Cass to a 4” Russian Maksutov? They’re both catadioptric (lens-mirror) systems, both mass-produced, and they’re both f/10. What could we learn?
|
|

|
|
|
|
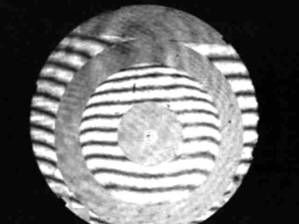
So the Maksutov went into the interferometer, too. The pictures of the C-8 are on the left, and the Mak pictures are on the right. First, a word about the setup. The light is running through the telescopes twice, making the scope’s wavefront errors appear twice as big as they really are. The fringes are 1/2 wave apart. That is, if you stretch a wire across the aperture, and the fringe above it just touches it, and the fringe below it just touches it, then the double-pass wavefront has a 1/2 wave error and the scope has a 1/4 wavefront error. This was a fast test, so the scopes were not in perfect thermal equilibrium (but what scope ever is?). The outer viewing circle is 6” in diameter. The big spot in the center of the interferogram is the hole made by the secondary. It looks bigger in the interferogram than on the actual corrector plate because it is bigger, because good baffling significantly enlarges the hole cut in the beam. Finally, once you get beyond the metal tube diameter on the Mak, you can see fringes made by a second flat behind the scope. They don’t mean anything. They are cut off at the top by the 2x4 I used to steady the Maksutov.
The first thing to notice is the fringes in the interferogram of the C-8. In the first picture, they curve down on the left side (that being the edge of the corrector plate). For most of the corrector plate (at least the part we can see), the fringes are generally straight, but the wavefront turns down by at least a quarter wave near the outer inch of aperture. This is, in Mr. Smith’s words, “double plus ungood,” because the outer edge makes up so much of the area of the scope, and makes so much of a contribution to the star’s image. When the edge turns down like this, the light is bent from its intended course, and ends up spreading out the star’s image point.
The next thing to notice is the fact that the fringes, while generally parallel, converge slightly toward the right. This is an indication of astigmatism, probably due to misaligned primary mirror in this telescope, and it can’t be corrected in commercial Schmidt Cassegrains, because the mirror is glued in place.
The next image has a wider fringe spacing, and it shows another type of error. Notice that the fringes have a “jaggy” appearance, and the jaggies are concentric about the secondary. These are faint ring zones, caused by fast polishing a nearly flat surface (the corrector plate). If you judge them by their heights, which is what we are measuring here, you might say that they are not serious defects, being a small fraction of a fringe spacing in height. However, they are very serious. They have a very high frequency, meaning that there are a lot of them in a short distance. If you’ve ever considered the ability of a fresnel lens, with its short height variations and lots of them per inch, to bend light out of the straight and narrow, then you might get some idea of the harm these ring zones do. Be cautious about wavefront claims, because this scope’s peak-to-valley errors are about 1/3 wave, and its RMS errors are about 1/12 wave. As commercial Schmidt Cassegrains go, this one is probably in the top third of all that I have seen, and I have never seen an Airy disk in this telescope.
The first Maksutov photo shows similar wavefront errors, including astigmatism, but it lacks the C-8’s turned down edge and the jaggies. The fringes are much cleaner and smoother. The second Mak photo (taken first) shows this better, but also shows thermal errors (bent fringes) near the inside and outside edges of the tube, and therefore doesn’t show the wavefront fairly.
The Maksutov, with all spherical surfaces, can be made more easily, and therefore more cheaply, than a Schmidt Cassegrain. This particular one gives better images, too.